This article will show you how to calculate Intraclass Correlation Coefficient (ICC) in Excel.
What is the Intraclass Correlation Coefficient?
The Intraclass Correlation Coefficient (ICC) determines the reliability of ratings for multiple items where there are multiple raters involved. The ratings are measured by comparing the variability of different ratings of the same item (or subject) rated reliably by different raters.
The ratings are quantitative. The value of an ICC can range from 0 to 1, where 0 means no reliability and 1 means perfect reliability among raters.
Based on the following three factors, the result of the various versions of an ICC is calculated.
| Factors | Description |
|---|---|
| Model |
|
| Type of Relationship |
|
| Unit |
|
How to Calculate Intraclass Correlation Coefficient (ICC) with Example
From this section, you will learn the steps for calculating ICC with an easy example.
Suppose four different judges were asked to rate the quality of 10 different products. The ratings given by them are shown below (and the example dataset for this article).
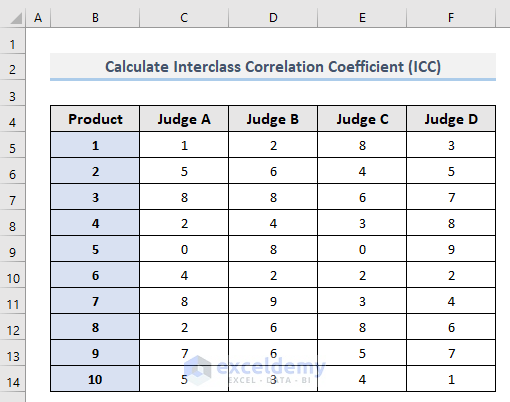
Now let’s learn the steps of calculating the ICC for this rating variability in Excel.
Steps:
- Firstly, click the tab Data -> Data Analysis.
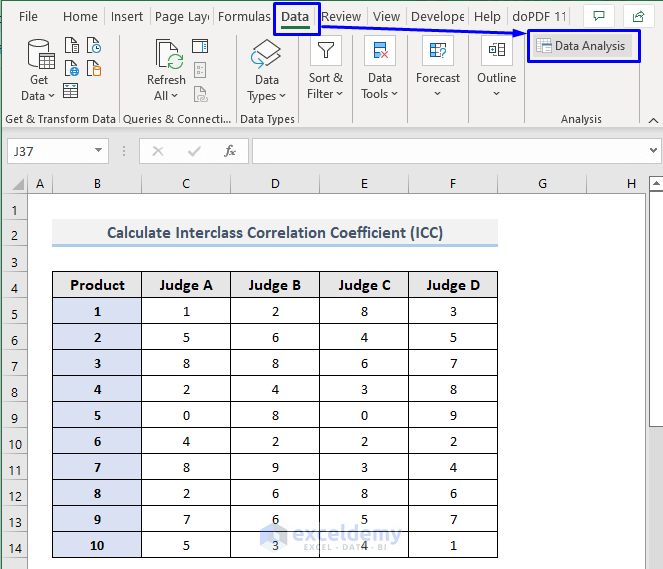
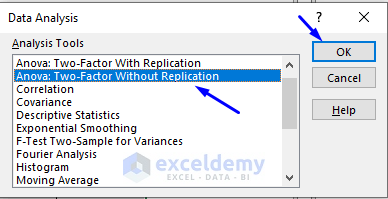
- Secondly, a Data Analysis pop-up window will appear. Then, select Anova: Two-Factor Without Replication.
- Later, click OK.
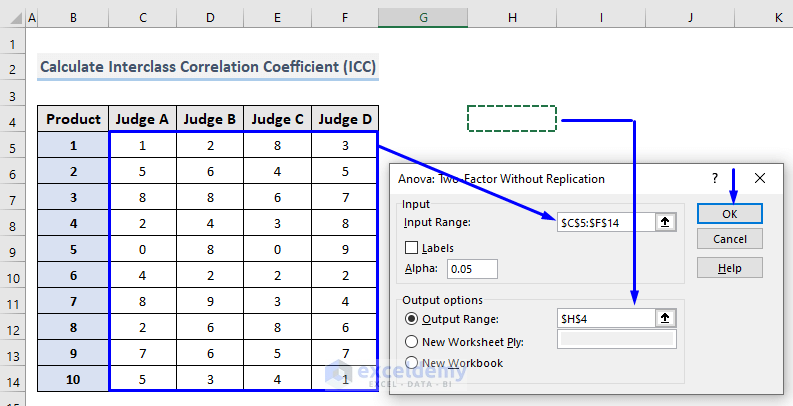
- After that, another Anova: Two-Factor Without Replication pop-up window will come up. At first, in the Input Range box, drag through the whole range from your dataset that contains the rating values. For our case, the range is C5:F14.
- Then, in the Output Range box, click on the cell in your dataset that you want as the starting cell of the output result. We want cell H4 as the starting cell of the output range, so we selected cell H4.
- Lastly, click OK.
- Finally, you will get the outcome of the Anova: Two-Factor Without Replication in your worksheet. Accordingly, you will be able to see the whole Summary of the calculations along with the individual Count, Sum, Average, and Variance of the ratings by the Judges for the Products.
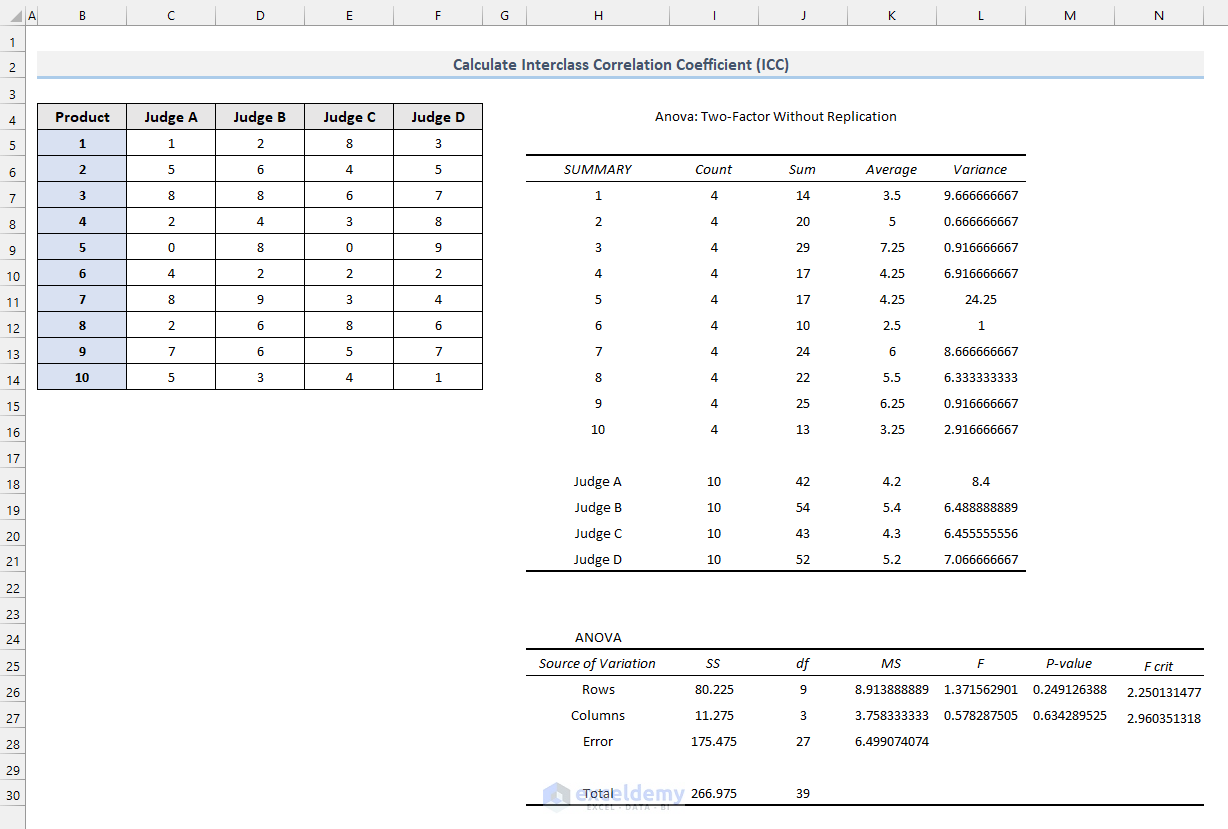
Then, to measure the ICC based on those rating variations, you need the bottom Anova table for input values.
The formula to calculate the Intraclass Correlation Coefficient (ICC) for the extracted output is:
=(K26-K28)/(K26+J27*K28+(J27+1)*(K27-K28)/(J26+1))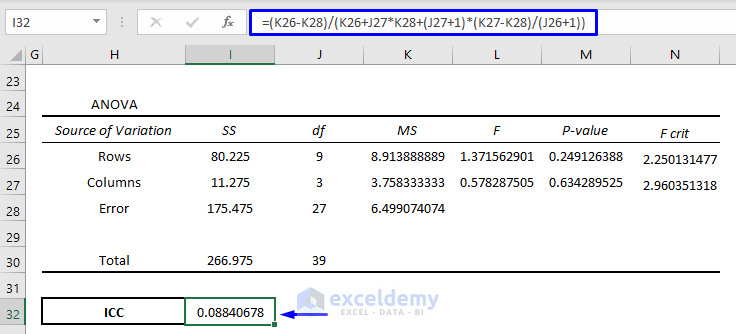
Subsequently, the Intraclass Correlation Coefficient (ICC) for our dataset is 0.08840678.
The interpretation of the value of an Intraclass Correlation Coefficient (ICC) is as follows:
- Less than 0.50 -> Poor reliability
- Between 0.5 and 0.75 -> Moderate reliability
- Between 0.75 and 0.9 -> Good reliability
- Greater than 0.9 -> Excellent reliability
As a result, we can conclude by saying that the calculated ICC of 0.08840678 refers to the products being rated with “poor reliability” by different raters.
Read More: How to Calculate Spearman Correlation in Excel
Download Workbook
You can download the free practice Excel workbook from here.
Conclusion
To conclude, this article showed you how to calculate Intraclass Correlation Coefficient (ICC) in Excel. Therefore, I hope this article has been very beneficial to you. Furthermore, feel free to ask if you have any questions regarding the topic.
Related Articles
- How to Calculate P Value for Spearman Correlation in Excel
- How to Show Relationship Between Two Variables in Excel Graph
- How to Calculate Correlation Coefficient in Excel
- How to Calculate Pearson Correlation Coefficient in Excel
- How to Find Coefficient of Determination in Excel
- How to Find Correlation Coefficient in Excel Scatter Plot
- How to Find Spearman Rank Correlation Coefficient in Excel
